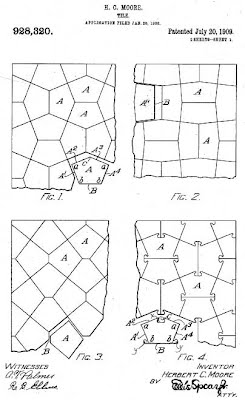
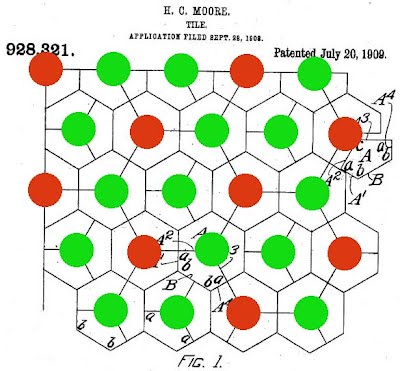
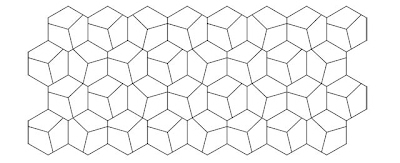
Introduction
In regards of my long-standing interest in convex pentagon tilings (and in particular the well-known Cairo tiling), I have recently been searching through US patents (these being the only instances that are readily searchable) for any instances of the genre. Surprisingly perhaps, there is very little of note, in both frequency and depth, and indeed there are only three patents worth considering, two by Herbert C. Moore (below), and one by Marc G. Odier. Of perhaps most note are the two by Moore, both of 1909, namely 928,320 and 928,321, the latter the subject of this page. The first and second patent shows what has subsequently become known as the ‘Cairo tiling’, and of which is to be a feature to my Cairo tiling pages, and so is not discussed here. The second patent has a different pentagonal tile, Fig. 1 (below) formed by subdividing a regular hexagon, or at least this is the easiest and most straightforward interpretation. As an aside, both patents are the earliest documented appearance to these two pentagonal tilings. And furthermore, Moore’s work predates any work in the field, this generally credited to Karl Reinhardt (in a thesis) in 1919, of which I detail below. This page examines Moore’s background, an analysis of this pentagon, much more deeper than Moore's account, investigates colouring possibilities. It also includes a most interesting in situ instance in Australia, and a variation of which can be titled as a ‘Reinhardt variation’.

Moore Patent

Moore Background
Of interest would be to know of Moore’s background – was he a mathematician or not? I do not know of any papers by him. The two instances above are his apparent entire work in the field, or at least as I have been able to find. Upon attempting to investigate his background, whether he was indeed a mathematician seems most unlikely. First, the only detail about him in both patents is his place of residence, namely Somerville, county of Middlesex, Commonwealth of Massachussets. No other biographical detail is given, and so this offers no clues. However, there are more exact details in the US 1900 census (with ages at the time of the census in brackets). Moore (37) was born in March 1863, in Maine, with his home in 1900 as Somerville Ward 4, Middlesex, Massachusetts. He was married to Annie F. Moore (31), and had a son, William H. Moore (9). Another member of the household was Annie Gordon (23), although there is no obvious family connection. In all instances the middle name letter is all that is given. In the census, he is described as a salesman, and although further detail as to this entry is given, the (handwritten) text is illegible. No mathematical background is discernible. Furthermore, he also has one other patent a few years later, of 1915, that differs widely from mathematics, on a ‘portiére or curtain hanger’ (the same details as to residence above are given). From this, although admittedly far from conclusive details, it would appear that he was not a mathematician but rather an amateur inventor in his spare time. Although most unlikely given the passage of time, I would be indebted to any reader who could provide any further biographical details of him, no matter how minor this may be thought. Underpinning this research is the possibility of him having undertook further work in the field, and whether his papers have survived.

Moore Pentagon

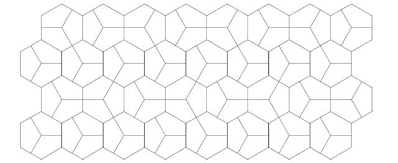
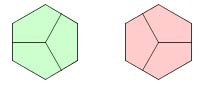
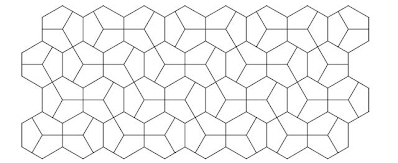
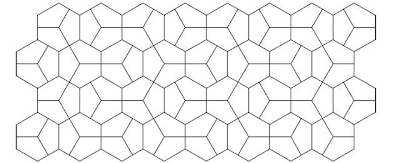
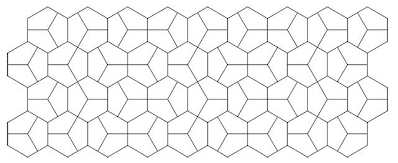
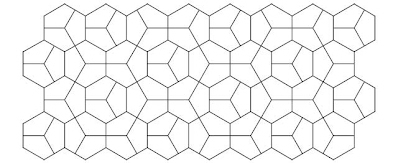
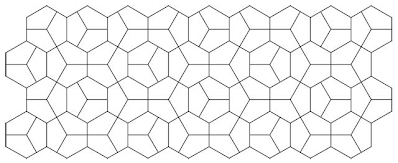
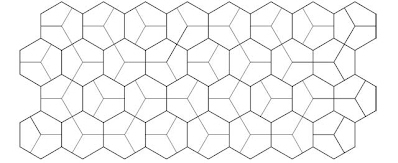
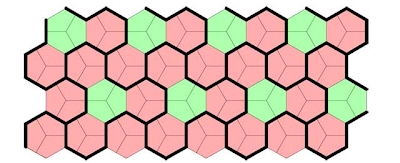
As this is the apparent first recorded instance of this tiling (does any reader know of an earlier example?), I now propose to honour Moore by describing this as a ‘Moore pentagon’. Moore shows but a single tiling, although there is much more to than this. Regarding the pentagonal tiling shown, this can be described as consisting of a regular hexagon and subdividing into three symmetrical pentagons, with sides of three different lengths. Another way of looking at this is of an order three ‘spoke’, the ends meeting on the mid point of a regular hexagon. Of interest is that the ‘spoke’ can be ‘placed’ inside the hexagon in two different ways, thus giving six different orientations of the pentagon.

Moore’s tiling show a combination as described above. Such a combining adds more interest to the possibilities. As might be imagined, with two possible placements, more than a single tiling is possible, of which Moore, although he must have been familiar with the possibilities does not discuss. Indeed, despite this being so obvious, he does not even mention anything about further tilings, or indeed hexagons! Moore’s two tilings (1909) is of significance historically, of which I believe this to be the first such ‘proper’ study of pentagon tilings, albeit the treatment is indeed lightweight. Moore predates Reinhardt by nine years, although their respective efforts are not to be compared in depth, with Reinhardt being incomparably the better mathematician, and looking at the problem in depth. Moore’s contribution is most limited, of two ad hoc instances, without thought as an all-encompassing approach. Nonetheless, Moore deserves much credit for being the first to at least look at pentagon tilings. I am unfamiliar to any reference to Moore in the pentagon tiling literature; his work seems to have been ignored, likely due to the means of his patent publication (although referred to in Sir Roger Penrose’s now famous Penrose tile patent of 1975), of which only recently, in the internet age, has such patent searching become practical. Does any reader know of any earlier instances of convex pentagon studies?

Studies

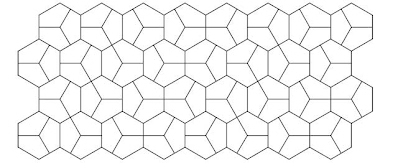
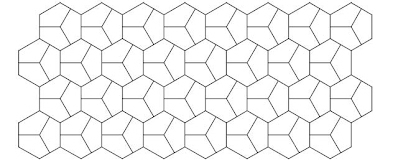
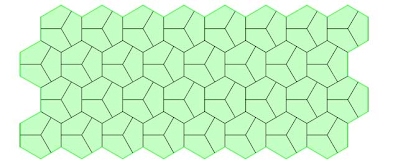
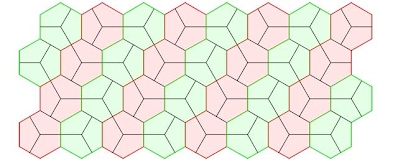
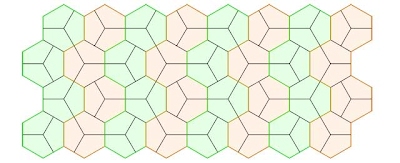
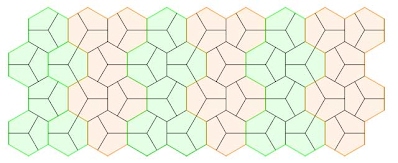
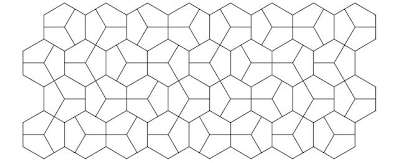
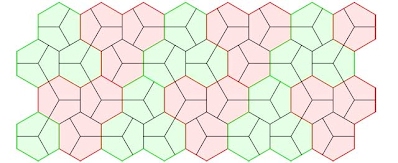
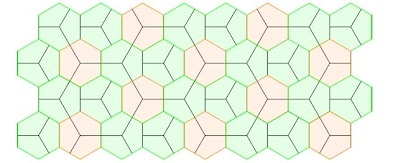
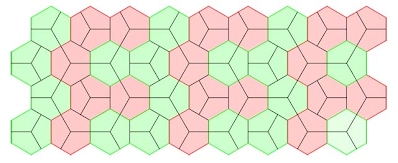
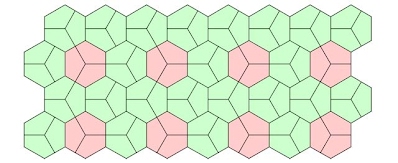
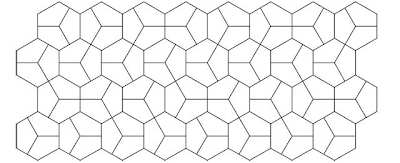
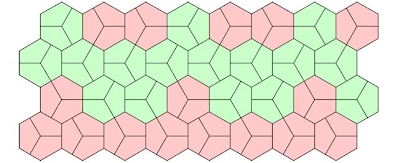
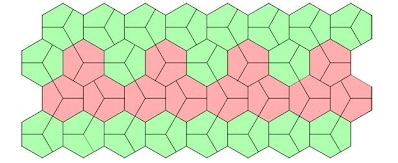
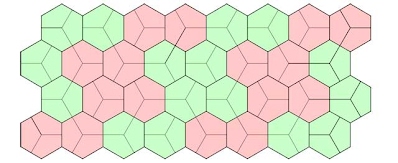
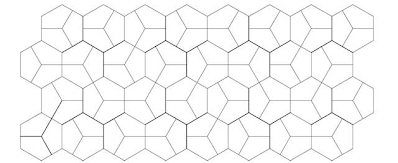
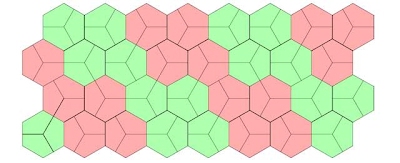
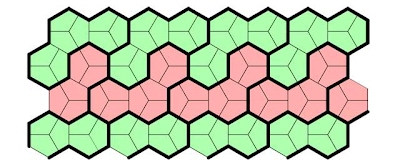
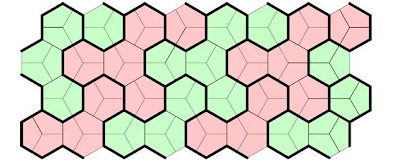
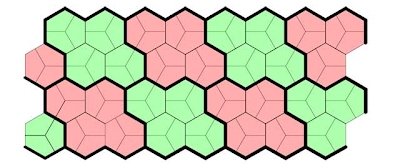
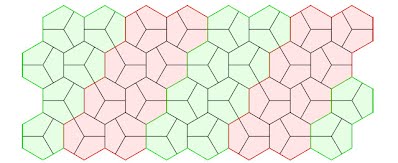
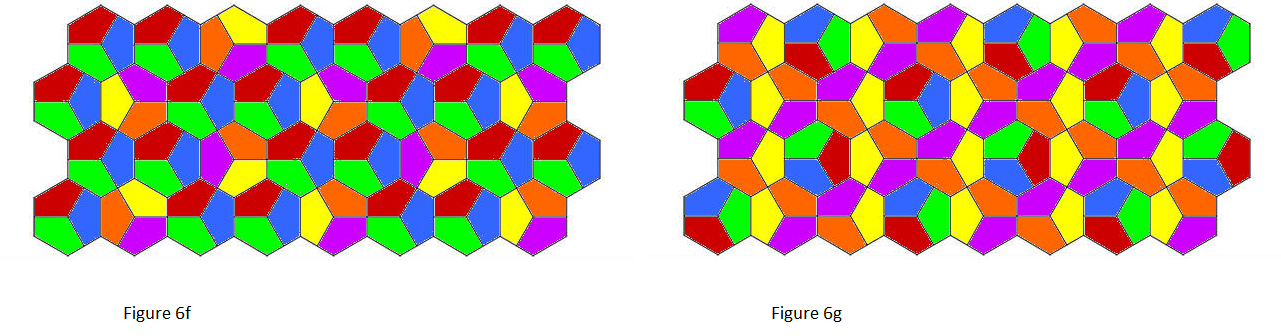
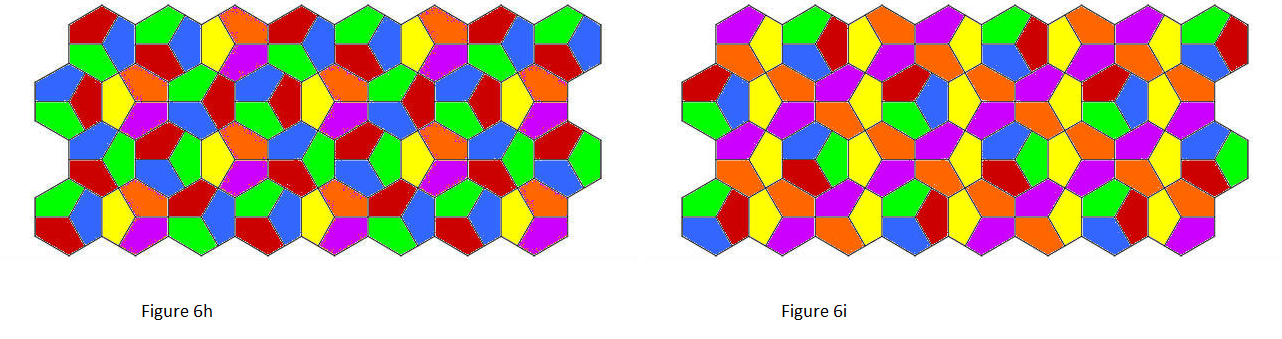
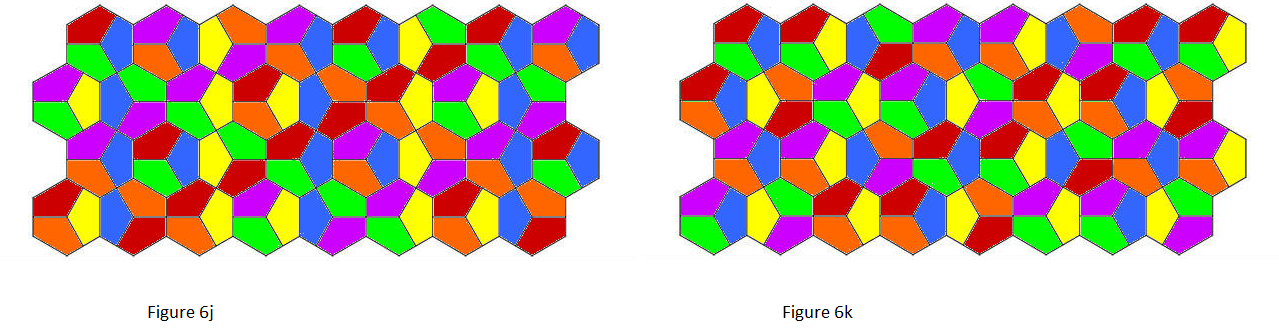
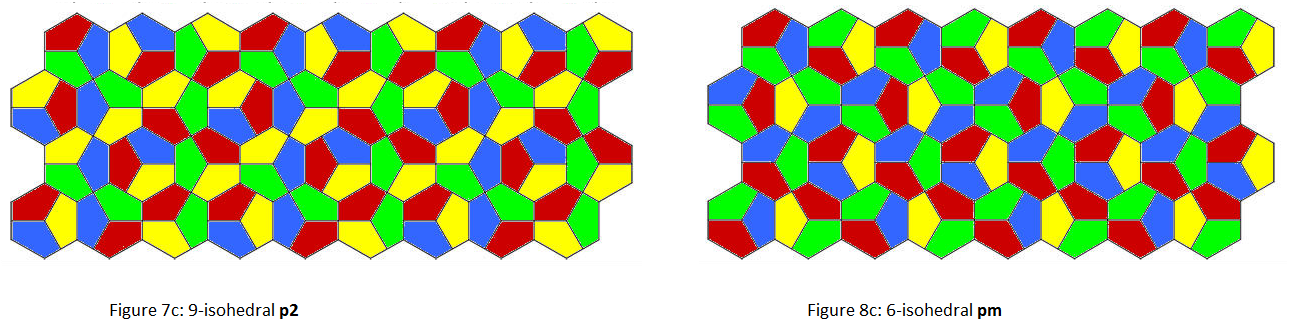
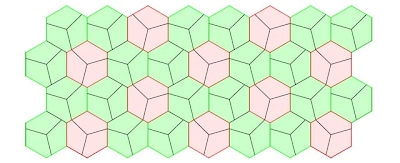
Upon correspondence with George Baloglou, with an shared interest in pentagon tiling, in which upon myself bringing this to his attention he urged me to study this tiling, I have recently looked at the possibilities, and of which I have drawn two main findings:
1. The pentagon tiles only in this hexagonal arrangement, i.e. all tilings have this underlying structure.
2. It can be readily seen that there are an infinity of tilings, by simply changing the ‘spoke’ in a mooted tiling at whim.

Consequently, of perhaps the most interest is to determine a number of non-trivial ‘core’ examples, some of which I show below, beginning with the simplest, and gradually increasing in complexity. The definition of ‘core’ possibilities is left in a loose, ill-defined state; there is no hard dividing line, I could continue with further tilings. These are in two forms: left, a wireframe diagram, and right, a colour-coded construction, based on the two placements of the spoke. This brings out the underlying structure much better. These are all shown in a relatively small format, of a linear block in an 8 x 4 array, as judged best to better show the construction in a ‘balanced’ manner.

Bailey Analysis